World Class Sprints

Part 3: Parameter Values

We turn now to the question of appropriate values for the parameters 20 years after Keller's work. The following tables record 1993 world-class performances in the 100 meter sprint, separately for men and women. Each table shows "split times" for 30, 60, 80, and 100 meters.

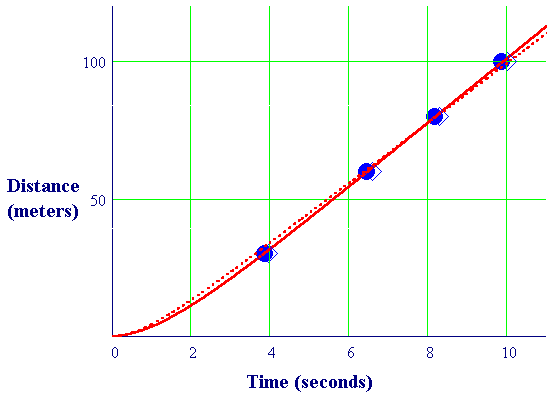
To illustrate the meanings of split times -- and the possibility that Keller's parameters are outdated -- we show the men's split times in the following figure, along with possible model distance functions. Christie's data (fastest) are shown as solid circles, and Lewis's (slowest) as open diamonds. The broken curve shows the distance function you calculated in Part 2 with Keller's parameters. The solid curve shows a possibly better fit -- with a faster terminal velocity.

1. Calculate average split times for men for each of the distances. (We will take up the women's data later.)
2. Each (time,distance) pair gives you numbers to substitute into your distance formula to get equations that involve only A and b. If you take ratios of two distances, say D₁ and D₂, you will have an equation that involves only b. Why? If the corresponding times are T₁ and T₂, explain why the resulting equation is
   

3. Solve the equation in step 2 for b, using any two (time,distance) pairs for the men. Then vary the (time,distance) pairs several times to get your best estimate of the parameter b for the men.
4. Explain why A can now be found from the equation
   

5. Solve for the parameter A for the men, again using several choices of (time,distance) pair to get your best estimate.
6. As a check on your work, plot your distance function for men together with the data for one of the men (or the average data, if you prefer).
7. Repeat steps 2 and 4 to estimate the parameters b and A for women.
8. Repeat step 5 with the women's distance function and data.