Inverse Functions

Part 1: Review: Exponential and Logarithm Functions

1. The following figure shows the graphs of y = e^x (red) and y = ln x (blue). Examine the graphs of these two functions, and describe the similarities and differences.
   

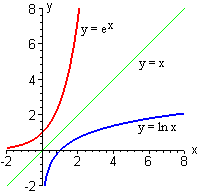
2. Describe a geometrical transformation that will take one graph into the other.

3. Explain your transformation algebraically in terms of interchange of the variables x and y. In what sense are the equations y = e^x and x = ln y equivalent?

4. In your worksheet, define f(x) = e^x and g(x) = ln x. Select a number of values of x, and calculate both g(f(x)) and f(g(x)). Explain your results.

5. Study the graphs of g( f (x)) and f (g(x)). Explain what you see. Why are the domains of the two functions different? [Warning: Your computer algebra system may not know that the domains of these functions are different. You are responsible for a correct mathematical answer even if the computer result is misleading. You don't have to explain what the CAS is doing -- just explain the mathematics.]

6. What does it mean to say that the natural exponential function and the natural logarithmic function are inverses?